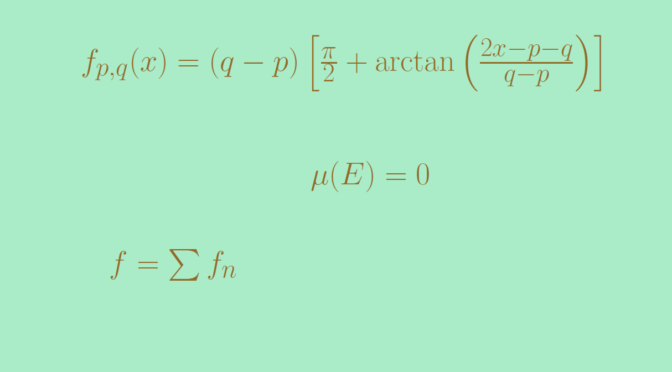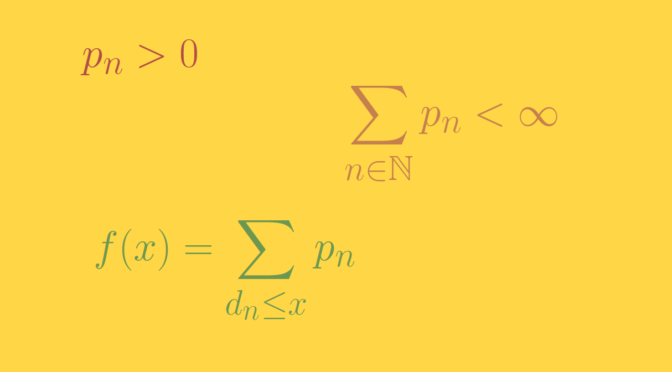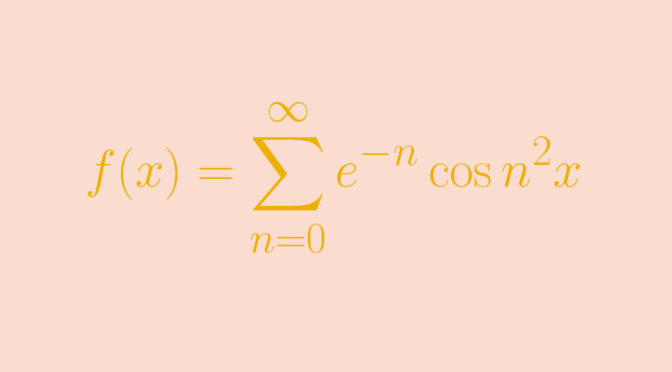Tag Archives: analysis

Analysis

A semi-continuous function with a dense set of points of discontinuity

Leave a comment

Let’s come back to Thomae’s function which is defined as:
\[f:
\left|\begin{array}{lrl}
\mathbb{R} & \longrightarrow & \mathbb{R} \\
x & \longmapsto & 0 \text{ if } x \in \mathbb{R} \setminus \mathbb{Q} \\
\frac{p}{q} & \longmapsto & \frac{1}{q} \text{ if } \frac{p}{q} \text{ in lowest terms and } q > 0
\end{array}\right.\]

We proved here that \(f\) right-sided and left-sided limits vanish at all points. Therefore \(\limsup\limits_{x \to a} f(x) \le f(a)\) at every point \(a\) which proves that \(f\) is upper semi-continuous on \(\mathbb R\). However \(f\) is continuous at all \(a \in \mathbb R \setminus \mathbb Q\) and discontinuous at all \(a \in \mathbb Q\).

Analysis

Converse of fundamental theorem of calculus

Leave a comment

The fundamental theorem of calculus asserts that for a continuous real-valued function \(f\) defined on a closed interval \([a,b]\), the function \(F\) defined for all \(x \in [a,b]\) by
\[F(x)=\int _{a}^{x}\!f(t)\,dt\] is uniformly continuous on \([a,b]\), differentiable on the open interval \((a,b)\) and \[
F^\prime(x) = f(x)\]
for all \(x \in (a,b)\).

The converse of fundamental theorem of calculus is not true as we see below.

Consider the function defined on the interval \([0,1]\) by \[
f(x)= \begin{cases}
2x\sin(1/x) – \cos(1/x) & \text{ for } x \neq 0 \\
0 & \text{ for } x = 0 \end{cases}\] \(f\) is integrable as it is continuous on \((0,1]\) and bounded on \([0,1]\). Then \[
F(x)= \begin{cases}
x^2 \sin \left( 1/x \right) & \text{ for } x \neq 0 \\
0 & \text{ for } x = 0 \end{cases}\] \(F\) is differentiable on \([0,1]\). It is clear for \(x \in (0,1]\). \(F\) is also differentiable at \(0\) as for \(x \neq 0\) we have \[
\left\vert \frac{F(x) – F(0)}{x-0} \right\vert = \left\vert \frac{F(x)}{x} \right\vert \le \left\vert x \right\vert.\] Consequently \(F^\prime(0) = 0\).

However \(f\) is not continuous at \(0\) as it does not have a right limit at \(0\).

Analysis

Counterexamples around series (part 2)

Leave a comment

We follow the article counterexamples around series (part 1) providing additional funny series examples.

If \(\sum u_n\) converges and \((u_n)\) is non-increasing then \(u_n = o(1/n)\)?

This is true. Let’s prove it.
The hypotheses imply that \((u_n)\) converges to zero. Therefore \(u_n \ge 0\) for all \(n \in \mathbb N\). As \(\sum u_n\) converges we have \[
\displaystyle \lim\limits_{n \to \infty} \sum_{k=n/2}^{n} u_k = 0.\] Hence for \(\epsilon \gt 0\), one can find \(N \in \mathbb N\) such that \[
\epsilon \ge \sum_{k=n/2}^{n} u_k \ge \frac{1}{2} (n u_n) \ge 0\] for all \(n \ge N\). Which concludes the proof.

\(\sum u_n\) convergent is equivalent to \(\sum u_{2n}\) and \(\sum u_{2n+1}\) convergent?

Is not true as we can see taking \(u_n = \frac{(-1)^n}{n}\). \(\sum u_n\) converges according to the alternating series test. However for \(n \in \mathbb N\) \[
\sum_{k=1}^n u_{2k} = \sum_{k=1}^n \frac{1}{2k} = 1/2 \sum_{k=1}^n \frac{1}{k}.\] Hence \(\sum u_{2n}\) diverges as the harmonic series diverges.

\(\sum u_n\) absolutely convergent is equivalent to \(\sum u_{2n}\) and \(\sum u_{2n+1}\) absolutely convergent?

This is true and the proof is left to the reader.

\(\sum u_n\) is a positive convergent series then \((\sqrt[n]{u_n})\) is bounded?

Is true. If not, there would be a subsequence \((u_{\phi(n)})\) such that \(\sqrt[\phi(n)]{u_{\phi(n)}} \ge 2\). Which means \(u_{\phi(n)} \ge 2^{\phi(n)}\) for all \(n \in \mathbb N\) and implies that the sequence \((u_n)\) is unbounded. In contradiction with the convergence of the series \(\sum u_n\).

If \((u_n)\) is strictly positive with \(u_n = o(1/n)\) then \(\sum (-1)^n u_n\) converges?

It does not hold as we can see with \[
u_n=\begin{cases} \frac{1}{n \ln n} & n \equiv 0 [2] \\
\frac{1}{2^n} & n \equiv 1 [2] \end{cases}\] Then for \(n \in \mathbb N\) \[
\sum_{k=1}^{2n} (-1)^k u_k \ge \sum_{k=1}^n \frac{1}{2k \ln 2k} – \sum_{k=1}^{2n} \frac{1}{2^k} \ge \sum_{k=1}^n \frac{1}{2k \ln 2k} – 1.\] As \(\sum \frac{1}{2k \ln 2k}\) diverges as can be proven using the integral test with the function \(x \mapsto \frac{1}{2x \ln 2x}\), \(\sum (-1)^n u_n\) also diverges.

Analysis

A nonzero continuous map orthogonal to all polynomials

1 Comment

Let’s consider the vector space \(\mathcal{C}^0([a,b],\mathbb R)\) of continuous real functions defined on a compact interval \([a,b]\). We can define an inner product on pairs of elements \(f,g\) of \(\mathcal{C}^0([a,b],\mathbb R)\) by \[
\langle f,g \rangle = \int_a^b f(x) g(x) \ dx.\]

It is known that \(f \in \mathcal{C}^0([a,b],\mathbb R)\) is the always vanishing function if we have \(\langle x^n,f \rangle = \int_a^b x^n f(x) \ dx = 0\) for all integers \(n \ge 0\). Let’s recall the proof. According to Stone-Weierstrass theorem, for all \(\epsilon >0\) it exists a polynomial \(P\) such that \(\Vert f – P \Vert_\infty \le \epsilon\). Then \[
\begin{aligned}
0 &\le \int_a^b f^2 = \int_a^b f(f-P) + \int_a^b fP\\
&= \int_a^b f(f-P) \le \Vert f \Vert_\infty \epsilon(b-a)
\end{aligned}\] As this is true for all \(\epsilon > 0\), we get \(\int_a^b f^2 = 0\) and \(f = 0\).

We now prove that the result becomes false if we change the interval \([a,b]\) into \([0, \infty)\), i.e. that one can find a continuous function \(f \in \mathcal{C}^0([0,\infty),\mathbb R)\) such that \(\int_0^\infty x^n f(x) \ dx\) for all integers \(n \ge 0\). In that direction, let’s consider the complex integral \[
I_n = \int_0^\infty x^n e^{-(1-i)x} \ dx.\] \(I_n\) is well defined as for \(x \in [0,\infty)\) we have \(\vert x^n e^{-(1-i)x} \vert = x^n e^{-x}\) and \(\int_0^\infty x^n e^{-x} \ dx\) converges. By integration by parts, one can prove that \[
I_n = \frac{n!}{(1-i)^{n+1}} = \frac{(1+i)^{n+1}}{2^{n+1}} n! = \frac{e^{i \frac{\pi}{4}(n+1)}}{2^{\frac{n+1}{2}}}n!.\] Consequently, \(I_{4p+3} \in \mathbb R\) for all \(p \ge 0\) which means \[
\int_0^\infty x^{4p+3} \sin(x) e^{-x} \ dx =0\] and finally \[
\int_0^\infty u^p \sin(u^{1/4}) e^{-u^{1/4}} \ dx =0\] for all integers \(p \ge 0\) using integration by substitution with \(x = u^{1/4}\). The function \(u \mapsto \sin(u^{1/4}) e^{-u^{1/4}}\) is one we were looking for.

Analysis

Counterexamples around series (part 1)

1 Comment

The purpose of this article is to provide some basic counterexamples on real series. Counterexamples are provided as answers to questions.

Unless otherwise stated, \((u_n)_{n \in \mathbb{N}}\) and \((v_n)_{n \in \mathbb{N}}\) are two real sequences.

If \((u_n)\) is non-increasing and converges to zero then \(\sum u_n\) converges?

Is not true. A famous counterexample is the harmonic series \(\sum \frac{1}{n}\) which doesn’t converge as \[
\displaystyle \sum_{k=p+1}^{2p} \frac{1}{k} \ge \sum_{k=p+1}^{2p} \frac{1}{2p} = 1/2,\] for all \(p \in \mathbb N\).

If \(u_n = o(1/n)\) then \(\sum u_n\) converges?

Does not hold as can be seen considering \(u_n=\frac{1}{n \ln n}\) for \(n \ge 2\). Indeed \(\int_2^x \frac{dt}{t \ln t} = \ln(\ln x) – \ln (\ln 2)\) and therefore \(\int_2^\infty \frac{dt}{t \ln t}\) diverges. We conclude that \(\sum \frac{1}{n \ln n}\) diverges using the integral test. However \(n u_n = \frac{1}{\ln n}\) converges to zero. Continue reading Counterexamples around series (part 1) →

Analysis

Counterexamples on real sequences (part 3)

Leave a comment

This article is a follow-up of Counterexamples on real sequences (part 2).

Let \((u_n)\) be a sequence of real numbers.

If \(u_{2n}-u_n \le \frac{1}{n}\) then \((u_n)\) converges?

This is wrong. The sequence
\[u_n=\begin{cases} 0 & \text{for } n \notin \{2^k \ ; \ k \in \mathbb N\}\\
1- 2^{-k} & \text{for } n= 2^k\end{cases}\]
is a counterexample. For \(n \gt 2\) and \(n \notin \{2^k \ ; \ k \in \mathbb N\}\) we also have \(2n \notin \{2^k \ ; \ k \in \mathbb N\}\), hence \(u_{2n}-u_n=0\). For \(n = 2^k\) \[
0 \le u_{2^{k+1}}-u_{2^k}=2^{-k}-2^{-k-1} \le 2^{-k} = \frac{1}{n}\] and \(\lim\limits_{k \to \infty} u_{2^k} = 1\). \((u_n)\) does not converge as \(0\) and \(1\) are limit points.

If \(\lim\limits_{n} \frac{u_{n+1}}{u_n} =1\) then \((u_n)\) has a finite or infinite limit?

This is not true. Let’s consider the sequence
\[u_n=2+\sin(\ln n)\] Using the inequality \(
\vert \sin p – \sin q \vert \le \vert p – q \vert\)
which is a consequence of the mean value theorem, we get \[
\vert u_{n+1} – u_n \vert = \vert \sin(\ln (n+1)) – \sin(\ln n) \vert \le \vert \ln(n+1) – \ln(n) \vert\] Therefore \(\lim\limits_n \left(u_{n+1}-u_n \right) =0\) as \(\lim\limits_n \left(\ln(n+1) – \ln(n)\right) = 0\). And \(\lim\limits_{n} \frac{u_{n+1}}{u_n} =1\) because \(u_n \ge 1\) for all \(n \in \mathbb N\).

I now assert that the interval \([1,3]\) is the set of limit points of \((u_n)\). For the proof, it is sufficient to prove that \([-1,1]\) is the set of limit points of the sequence \(v_n=\sin(\ln n)\). For \(y \in [-1,1]\), we can pickup \(x \in \mathbb R\) such that \(\sin x =y\). Let \(\epsilon > 0\) and \(M \in \mathbb N\) , we can find an integer \(N \ge M\) such that \(0 < \ln(n+1) - \ln(n) \lt \epsilon\) for \(n \ge N\). Select \(k \in \mathbb N\) with \(x +2k\pi \gt \ln N\) and \(N_\epsilon\) with \(\ln N_\epsilon \in (x +2k\pi, x +2k\pi + \epsilon)\). This is possible as \((\ln n)_{n \in \mathbb N}\) is an increasing sequence and the length of the interval \((x +2k\pi, x +2k\pi + \epsilon)\) is equal to \(\epsilon\). We finally get \[ \vert u_{N_\epsilon} - y \vert = \vert \sin \left(\ln N_\epsilon \right) - \sin \left(x + 2k \pi \right) \vert \le \left(\ln N_\epsilon - (x +2k\pi)\right) \le \epsilon\] proving that \(y\) is a limit point of \((u_n)\).

Analysis

A strictly increasing continuous function that is differentiable at no point of a null set

Leave a comment

We build in this article a strictly increasing continuous function \(f\) that is differentiable at no point of a null set \(E\). The null set \(E\) can be chosen arbitrarily. In particular it can have the cardinality of the continuum like the Cantor null set.

A set of strictly increasing continuous functions

For \(p \lt q\) two real numbers, consider the function \[
f_{p,q}(x)=(q-p) \left[\frac{\pi}{2} + \arctan{\left(\frac{2x-p-q}{q-p}\right)}\right]\] \(f_{p,q}\) is positive and its derivative is \[
f_{p,q}^\prime(x) = \frac{2}{1+\left(\frac{2x-p-q}{q-p}\right)^2}\] which is always strictly positive. Hence \(f_{p,q}\) is strictly increasing. We also have \[
\lim\limits_{x \to -\infty} f_{p,q}(x) = 0 \text{ and } \lim\limits_{x \to \infty} f_{p,q}(x) = \pi(q-p).\] One can notice that for \(x \in (p,q)\), \(f_{p,q}^\prime(x) \gt 1\). Therefore for \(x, y \in (p,q)\) distinct we have according to the mean value theorem \(\frac{f_{p,q}(y)-f_{p,q}(x)}{y-x} \ge 1\).

Covering \(E\) with an appropriate set of open intervals

As \(E\) is a null set, for each \(n \in \mathbb N\) one can find an open set \(O_n\) containing \(E\) and measuring less than \(2^{-n}\). \(O_n\) can be written as a countable union of disjoint open intervals as any open subset of the reals. Then \(I=\bigcup_{m \in \mathbb N} O_m\) is also a countable union of open intervals \(I_n\) with \(n \in \mathbb N\). The sum of the lengths of the \(I_n\) is less than \(1\). Continue reading A strictly increasing continuous function that is differentiable at no point of a null set →

Analysis

A monotonic function whose points of discontinuity form a dense set

Leave a comment

Consider a compact interval \([a,b] \subset \mathbb R\) with \(a \lt b\). Let’s build an increasing function \(f : [a,b] \to \mathbb R\) whose points of discontinuity is an arbitrary dense subset \(D = \{d_n \ ; \ n \in \mathbb N\}\) of \([a,b]\), for example \(D = \mathbb Q \cap [a,b]\).

Let \(\sum p_n\) be a convergent series of positive numbers whose sum is equal to \(p\) and define \(\displaystyle f(x) = \sum_{d_n \le x} p_n\).

\(f\) is strictly increasing

For \(a \le x \lt y \le b\) we have \[
f(y) – f(x) = \sum_{x \lt d_n \le y} p_n \gt 0\] as the \(p_n\) are positive and dense so it exists \(p_m \in (x, y]\).

\(f\) is right-continuous on \([a,b]\)

We pick-up \(x \in [a,b]\). For any \(\epsilon \gt 0\) is exists \(N \in \mathbb N\) such that \(0 \lt \sum_{n \gt N} p_n \lt \epsilon\). Let \(\delta > 0\) be so small that the interval \((x,x+\delta)\) doesn’t contain any point in the finite set \(\{p_1, \dots, p_N\}\). Then \[
0 \lt f(y) – f(x) \le \sum_{n \gt N} p_n \lt \epsilon,\] for any \(y \in (x,x+\delta)\) proving the right-continuity of \(f\) at \(x\). Continue reading A monotonic function whose points of discontinuity form a dense set →

Analysis

A function whose Maclaurin series converges only at zero

Leave a comment

Let’s describe a real function \(f\) whose Maclaurin series converges only at zero. For \(n \ge 0\) we denote \(f_n(x)= e^{-n} \cos n^2x\) and \[
f(x) = \sum_{n=0}^\infty f_n(x)=\sum_{n=0}^\infty e^{-n} \cos n^2 x.\] For \(k \ge 0\), the \(k\)th-derivative of \(f_n\) is \[
f_n^{(k)}(x) = e^{-n} n^{2k} \cos \left(n^2 x + \frac{k \pi}{2}\right)\] and \[
\left\vert f_n^{(k)}(x) \right\vert \le e^{-n} n^{2k}\] for all \(x \in \mathbb R\). Therefore \(\displaystyle \sum_{n=0}^\infty f_n^{(k)}(x)\) is normally convergent and \(f\) is an indefinitely differentiable function with \[
f^{(k)}(x) = \sum_{n=0}^\infty e^{-n} n^{2k} \cos \left(n^2 x + \frac{k \pi}{2}\right).\] Its Maclaurin series has only terms of even degree and the absolute value of the term of degree \(2k\) is \[
\left(\sum_{n=0}^\infty e^{-n} n^{4k}\right)\frac{x^{2k}}{(2k)!} > e^{-2k} (2k)^{4k}\frac{x^{2k}}{(2k)!} > \left(\frac{2kx}{e}\right)^{2k}.\] The right hand side of this inequality is greater than \(1\) for \(k \ge \frac{e}{2x}\). This means that for any nonzero \(x\) the Maclaurin series for \(f\) diverges.

Analysis

Painter’s paradox

Leave a comment

Can you paint a surface with infinite area with a finite quantity of paint? For sure… let’s do it!

Consider the 3D surface given in cylindrical coordinates as \[
S(\rho,\varphi):\begin{cases}
x &= \rho \cos \varphi\\
y &= \rho \sin \varphi\\
z &= \frac{1}{\rho}\end{cases}\] for \((\rho,\varphi) \in [1,\infty) \times [0, 2 \pi)\). The surface is named Gabriel’s horn.

Volume of Gabriel’s horn

The volume of Gabriel’s horn is \[
V = \pi \int_1^\infty \left( \frac{1}{\rho^2} \right) \ d\rho = \pi\] which is finite.

Area of Gabriel’s horn

The area of Gabriel’s horn for \((\rho,\varphi) \in [1,a) \times [0, 2 \pi)\) with \(a > 1\) is: \[
A = 2 \pi \int_1^a \frac{1}{\rho} \sqrt{1+\left( -\frac{1}{\rho^2} \right)^2} \ d\rho \ge 2 \pi \int_1^a \frac{d \rho}{\rho} = 2 \pi \log a.\] As the right hand side of inequality above diverges to \(\infty\) as \(a \to \infty\), we can conclude that the area of Gabriel’s horn is infinite.

Conclusion

Gabriel’s horn could be filled with a finite quantity of paint… therefore painting a surface with infinite area. Unfortunately the thickness of the paint coat is converging to \(0\) as \(z\) goes to \(\infty\), leading to a paint which won’t be too visible!