Counterexample around Arzela-Ascoli theorem

June 7, 2015 Jean-Pierre Merx Leave a comment

Suppose that \(F\) is a Banach space and \(E\) a compact metric space. A subset \(\mathcal{H}\) of the Banach space \(\mathcal{C}_F(E)\) is relatively compact in the topology induced by the uniform norm if and only if it is equicontinuous and and for all \(x \in E\), the set \(\mathcal{H}(x)=\{f(x) \ | \ f \in \mathcal{H}\}\) is relatively compact.

We look here at what happens if we drop the requirement on space \(E\) to be compact and provide a counterexample where the conclusion of Arzelà–Ascoli theorem doesn’t hold anymore.

We take for \(E\) the real interval \([0,+\infty)\) and for all \(n \in \mathbb{N} \setminus \{0\}\) the real function
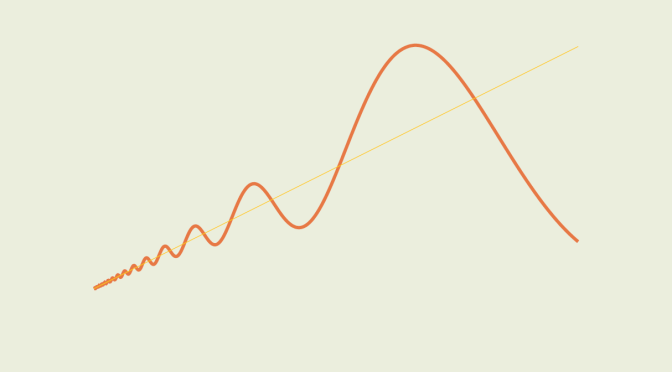
\[f_n(t)= \sin \sqrt{t+4 n^2 \pi^2}\] We prove that \((f_n)\) is equicontinuous, converges pointwise to \(0\) but is not relatively compact.

According to the mean value theorem, for all \(x,y \in \mathbb{R}\)
\[\vert \sin x – \sin y \vert \le \vert x – y \vert\] Hence for \(n \ge 1\) and \(x,y \in [0,+\infty)\)
\begin{align*}
\vert f_n(x)-f_n(y) \vert &\le \vert \sqrt{x+4 n^2 \pi^2} -\sqrt{y+4 n^2 \pi^2} \vert \\
&= \frac{\vert x – y \vert}{\sqrt{x+4 n^2 \pi^2} +\sqrt{y+4 n^2 \pi^2}} \\
&\le \frac{\vert x – y \vert}{4 \pi}
\end{align*} using multiplication by the conjugate.

Which enables to prove that \((f_n)\) is equicontinuous.

We also have for \(n \ge 1\) and \(x \in [0,+\infty)\)
\begin{align*}
\vert f_n(x) \vert &= \vert f_n(x) – f_n(0) \vert \le \vert \sqrt{x+4 n^2 \pi^2} -\sqrt{4 n^2 \pi^2} \vert \\
&= \frac{\vert x \vert}{\sqrt{x+4 n^2 \pi^2} +\sqrt{4 n^2 \pi^2}} \\
&\le \frac{\vert x \vert}{4 n \pi}
\end{align*}

Hence \((f_n)\) converges pointwise to \(0\) and for \(t \in [0,+\infty), \mathcal{H}(t)=\{f_n(t) \ | \ n \in \mathbb{N} \setminus \{0\}\}\) is relatively compact

Finally we prove that \(\mathcal{H}=\{f_n \ | \ n \in \mathbb{N} \setminus \{0\}\}\) is not relatively compact. While \((f_n)\) converges pointwise to \(0\), \((f_n)\) does not converge uniformly to \(f=0\). Actually for \(n \ge 1\) and \(t_n=\frac{\pi^2}{4} + 2n \pi^2\) we have
\[f_n(t_n)= \sin \sqrt{\frac{\pi^2}{4} + 2n \pi^2 +4 n^2 \pi^2}=\sin \sqrt{\left(\frac{\pi}{2} + 2 n \pi\right)^2}=1\] Consequently for all \(n \ge 1\) \(\Vert f_n – f \Vert_\infty \ge 1\). If \(\mathcal{H}\) was relatively compact, \((f_n)\) would have a convergent subsequence with \(f=0\) for limit. And that cannot be as for all \(n \ge 1\) \(\Vert f_n – f \Vert_\infty \ge 1\).

Analysis

Pointwise convergence and properties of the limit (part 1)

May 31, 2015 Jean-Pierre Merx Leave a comment

We look here at the continuity of a sequence of functions that converges pointwise and give some counterexamples of what happens versus uniform convergence.

Recalling the definition of pointwise convergence

We consider here real functions defined on a closed interval \([a,b]\). A sequence of functions \((f_n)\) defined on \([a,b]\) converges pointwise to the function \(f\) if and only if for all \(x \in [a,b]\) \(\displaystyle \lim\limits_{n \to +\infty} f_n(x) = f(x)\). Pointwise convergence is weaker than uniform convergence.

Pointwise convergence does not, in general, preserve continuity

Suppose that \(f_n \ : \ [0,1] \to \mathbb{R}\) is defined by \(f_n(x)=x^n\). For \(0 \le x <1\) then \(\displaystyle \lim\limits_{n \to +\infty} x^n = 0\), while if \(x = 1\) then \(\displaystyle \lim\limits_{n \to +\infty} x^n = 1\). Hence the sequence \(f_n\) converges to the function equal to \(0\) for \(0 \le x < 1\) and to \(1\) for \(x=1\). Although each \(f_n\) is a continuous function of \([0,1]\), their pointwise limit is not. \(f\) is discontinuous at \(1\). We notice that \((f_n)\) doesn't converge uniformly to \(f\) as for all \(n \in \mathbb{N}\), \(\displaystyle \sup\limits_{x \in [0,1]} \vert f_n(x) - f(x) \vert = 1\). That's reassuring as uniform convergence of a sequence of continuous functions implies that the limit is continuous! Continue reading Pointwise convergence and properties of the limit (part 1) →

Topology

A topological vector space with no non trivial continuous linear form

May 16, 2015 Jean-Pierre Merx Leave a comment

We consider here the \(L^p\)- spaces of real functions defined on \([0,1]\) for which the \(p\)-th power of the absolute value is Lebesgue integrable. We focus on the case \(0 < p < 1\). We'll prove that those \(L^p\)-spaces are topological vector spaces on which there exists no continuous non-trivial linear forms (i.e. not vanishing identically). Continue reading A topological vector space with no non trivial continuous linear form →

Analysis

A nowhere locally bounded function

May 15, 2015 Jean-Pierre Merx 1 Comment

In that article, I described some properties of Thomae’s function\(f\). Namely:

The function is discontinuous on \(\mathbb{Q}\).
Continuous on \(\mathbb{R} \setminus \mathbb{Q}\).
Its right-sided and left-sided limits vanish at all points.

Let’s modify \(f\) to get function \(g\) defined as follow:
\[g:
\left|\begin{array}{lrl}
\mathbb{R} & \longrightarrow & \mathbb{R} \\
x & \longmapsto & 0 \text{ if } x \in \mathbb{R} \setminus \mathbb{Q} \\
\frac{p}{q} & \longmapsto & q \text{ if } \frac{p}{q} \text{ in lowest terms and } q > 0
\end{array}\right.\] \(f\) and \(g\) both vanish on the set of irrational numbers, while on the set of rational numbers, \(g\) is equal to the reciprocal of \(f\). We now consider an open subset \(O \subset \mathbb{R}\) and \(x \in O\). As \(f\) right-sided and left-sided limits vanish at all points, we have \(\lim\limits_{n \to +\infty} f(x_n) = 0\) for all sequence \((x_n)\) of rational numbers converging to \(x\) (and such a sequence exists as the rational numbers are everywhere dense in the reals). Hence \(\lim\limits_{n \to +\infty} g(x_n) = + \infty\) as \(f\) is positive.

We can conclude that \(g\) is nowhere locally bounded. The picture of the article is a plot of function \(g\) on the rational numbers \(r = \frac{p}{q}\) in lowest terms for \(0 < r < 1\) and \(q \le 50\).

Analysis

A function continuous at all irrationals and discontinuous at all rationals

May 10, 2015 Jean-Pierre Merx 2 Comments

Let’s discover the beauties of Thomae’s function also named the popcorn function, the raindrop function or the modified Dirichlet function.

Thomae’s function is a real-valued function defined as:
\[f:
\left|\begin{array}{lrl}
\mathbb{R} & \longrightarrow & \mathbb{R} \\
x & \longmapsto & 0 \text{ if } x \in \mathbb{R} \setminus \mathbb{Q} \\
\frac{p}{q} & \longmapsto & \frac{1}{q} \text{ if } \frac{p}{q} \text{ in lowest terms and } q > 0
\end{array}\right.\]

\(f\) is periodic with period \(1\)

This is easy to prove as for \(x \in \mathbb{R} \setminus \mathbb{Q}\) we also have \(x+1 \in \mathbb{R} \setminus \mathbb{Q}\) and therefore \(f(x+1)=f(x)=0\). While for \(y=\frac{p}{q} \in \mathbb{Q}\) in lowest terms, \(y+1=\frac{p+q}{q}\) is also in lowest terms, hence \(f(y+1)=f(y)=\frac{1}{q}\). Continue reading A function continuous at all irrationals and discontinuous at all rationals →

Algebra

Generating the symmetric group with a transposition and a maximal length cycle

May 2, 2015 Jean-Pierre Merx Leave a comment

Can the symmetric group \(\mathcal{S}_n\) be generated by any transposition and any \(n\)-cycle for \(n \ge 2\) integer? is the question we deal with.

We first recall some terminology:

Symmetric group: The symmetric group \(\mathcal{S}_n\) on a finite set of \(n\) symbols is the group whose elements are all the permutations of the \(n\) symbols. We’ll denote by \(\{1,\dots,n\}\) those \(n\) symbols.
Cycle: A cycle of length \(k\) (with \(k \ge 2\)) is a cyclic permutation \(\sigma\) for which there exists an element \(i \in \{1,\dots,n\}\) such that \(i, \sigma(i), \sigma^2(i), \dots, \sigma^k(i)=i\) are the only elements moved by \(\sigma\). We’ll denote the cycle \(\sigma\) by \((s_0 \ s_1 \dots \ s_{k-1})\) where \(s_0=i, s_1=\sigma(i),\dots,s_{k-1}=\sigma^{k-1}(i)\).
Transposition: A transposition is a cycle of length \(2\). We denote below the transposition of elements \(a \neq b\) by \((a \ b)\) or \(\tau_{a,b}\).

Continue reading Generating the symmetric group with a transposition and a maximal length cycle →

Topology

Distance between a point and a hyperplane not reached

April 26, 2015 Jean-Pierre Merx Leave a comment

Let’s investigate the following question: “Is the distance between a point and a hyperplane always reached?”

In order to provide answers to the question, we consider a normed vector space \((E, \Vert \cdot \Vert)\) and a hyperplane \(H\) of \(E\). \(H\) is the kernel of a non-zero linear form. Namely, \(H=\{x \in E \text{ | } u(x)=0\}\).

The case of finite dimensional vector spaces

When \(E\) is of finite dimension, the distance \(d(a,H)=\inf\{\Vert h-a \Vert \text{ | } h \in H\}\) between any point \(a \in E\) and a hyperplane \(H\) is reached at a point \(b \in H\). The proof is rather simple. Consider a point \(c \in H\). The set \(S = \{h \in H \text{ | } \Vert a- h \Vert \le \Vert a-c \Vert \}\) is bounded as for \(h \in S\) we have \(\Vert h \Vert \le \Vert a-c \Vert + \Vert a \Vert\). \(S\) is equal to \(D \cap H\) where \(D\) is the inverse image of the closed real segment \([0,\Vert a-c \Vert]\) by the continuous map \(f: x \mapsto \Vert a- x \Vert\). Therefore \(D\) is closed. \(H\) is also closed as any linear subspace of a finite dimensional vector space. \(S\) being the intersection of two closed subsets of \(E\) is also closed. Hence \(S\) is compact and the restriction of \(f\) to \(S\) reaches its infimum at some point \(b \in S \subset H\) where \(d(a,H)=\Vert a-b \Vert\). Continue reading Distance between a point and a hyperplane not reached →

Analysis

A function whose derivative at 0 is one but which is not increasing near 0

April 19, 2015 Jean-Pierre Merx Leave a comment

From the mean value theorem, a real function whose derivative is strictly positive at every point of an interval is strictly increasing. In particular, a continuously differentiable function \(f\) defined in a non-degenerate interval \(I\) with a strictly positive derivative at a point \(a\) of the interval is strictly increasing near that point. For the proof, we just have to notice that as \(f^\prime\) is continuous and \(f^\prime(a) > 0\), \(f^\prime\) is strictly positive within an interval \(J \subset I\) containing \(a\). By the mean value theorem, \(f\) is strictly increasing on \(J\).

We now suppose that \(f\) is differentiable on an interval \(I\) containing \(0\) with \(f^\prime(0)>0\). For \(x>0\) sufficiently close to zero we have \(\displaystyle \frac{f(x)-f(0)}{x-0} > \frac{f^\prime(0)}{2}>0\), hence \(f(x)>f(0)\). But that doesn’t imply that \(f\) is strictly increasing in a neighborhood of zero. Let’s prove it with a counterexample. Continue reading A function whose derivative at 0 is one but which is not increasing near 0 →

Math Counterexamples

Counterexample around Arzela-Ascoli theorem

Pointwise convergence and properties of the limit (part 1)

Recalling the definition of pointwise convergence

Pointwise convergence does not, in general, preserve continuity

A topological vector space with no non trivial continuous linear form

A nowhere locally bounded function

A function continuous at all irrationals and discontinuous at all rationals

\(f\) is periodic with period \(1\)

Generating the symmetric group with a transposition and a maximal length cycle

Distance between a point and a hyperplane not reached

The case of finite dimensional vector spaces

A function whose derivative at 0 is one but which is not increasing near 0

Mathematical exceptions to the rules or intuition

\(\mathbb{Z}_{p^\infty}\) is a group

Recalling the definition of pointwise convergence

Pointwise convergence does not, in general, preserve continuity

Description of the group \(G\)

\(f\) is periodic with period \(1\)

The case of finite dimensional vector spaces

Mathematical exceptions to the rules or intuition