Let’s recall Lebesgue’s Dominated Convergence Theorem. Let \((f_n)\) be a sequence of real-valued measurable functions on a measure space \((X, \Sigma, \mu)\). Suppose that the sequence converges pointwise to a function \(f\) and is dominated by some integrable function \(g\) in the sense that \[
\vert f_n(x) \vert \le g (x)\] for all \(n \in \mathbb N\) and all \(x \in X\).
Then \(f\) is integrable and \[
\lim\limits_{n \to \infty} \int_X f_n(x) \ d \mu = \int_X f(x) \ d \mu\]

Let’s see what can happen if we drop the domination condition.

We consider the space \(\mathbb R\) endowed with Lebesgue measure and for \(E \subseteq \mathbb R\) we denote by \(\chi_E\) the indicator function of \(E\) defined by \[
\chi_E(x)=\begin{cases}
1 \text{ if } x \in E\\
0 \text{ otherwise}\end{cases}\] For \(n \in \mathbb N\), the function \(f_n=\frac{1}{2n}\chi_{(n^2-n,n^2+n)}\) is measurable and we have \[
\int_{\mathbb R} \frac{1}{2n}\chi_{(n^2-n,n^2+n)}(x) \ dx = \int_{n^2-n}^{n^2+n} \frac{1}{2n} \ dx = 1\] The sequence \((f_n)\) converges uniformly (and therefore pointwise) to the always vanishing function as for \(n \in \mathbb N\) we have for all \(x \in \mathbb R\) \(\vert f_n(x) \vert \le \frac{1}{2n}\). Hence the conclusion of Lebesgue’s Dominated Convergence Theorem doesn’t hold for the sequence \((f_n)\).

Let’s verify that the sequence \((f_n)\) is not dominated by some integrable function \(g\). For \(p < q\) integers, we have \[ \begin{aligned} q^2-q-(p^2+p) &= q^2-p^2 -q-p\\ &= (q-p)(q+p) -q -p\\ &\ge (q+p) -q-p=0 \end{aligned}\] Hence for \(p \neq q\) integers the intervals \((p^2-p,p^2+p)\) and \((q^2-q,q^2+q)\) are disjoint. Consequently for all \(x \in \mathbb R\) the sum \(\sum_{n \in \mathbb N} f_n(x)\) amounts to only one term and the function \(\sum_{n \in \mathbb N} f_n\) is well defined. If \(g\) dominates the sequence \((f_n)\), it satisfies \(0 \le \sum_{n \in \mathbb N} f_n \le g\). But \[ \int_{\mathbb R} \sum_{n \in \mathbb N} f_n(x) \ dx = \sum_{n \in \mathbb N} \int_{\mathbb R} f_n(x) \ dx = \sum_{n \in \mathbb N} 1 = \infty\] and \(g\) cannot be integrable.

The case of a nowhere converging function

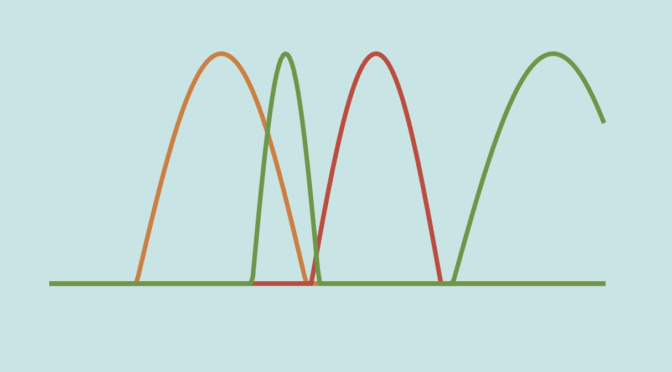
Let’s now construct a sequence of continuous functions \(g_n\) defined on \( [0, 1]\) such that \(0 \le g_n \le 1\) and \[
\lim\limits_{n \to \infty} \int_0^1 g_n(x) \ dx = 0,\] but such that the sequence \((g_n(x))\) converges for no \(x \in [0,1]\).

Consider first the continuous and non negative function \(c : \mathbb R \to [0,1]\) defined by \[
c(x) = \begin{cases}
\cos \frac{\pi x}{2} &\text{for } x \in [-1,1]\\
0 &\text{otherwise}
\end{cases}\] We have \[
\int_{\mathbb R} c(x) \ dx = \int_{-1}^1 \cos \frac{\pi x}{2} \ dx = \frac{4}{\pi}\]

Let’s also denote by \((r_n)\) the sequence whose initial terms are \[
\frac{1}{2}, \frac{1}{3}, \frac{2}{3}, \frac{1}{4}, \frac{2}{4}, \frac{3}{4}, \frac{1}{5}, \frac{2}{5}, \frac{3}{5}, \frac{4}{5}, \frac{1}{6}, \frac{2}{6}, \frac{3}{6}, \frac{4}{6}, \frac{5}{6}, \dots\] and whose formal definition is \[
r_n=\begin{cases}
\frac{1}{2} &\text{ for } n= 1\\
\frac{n}{k+2} – \frac{k(k+1)}{2(k+2)} &\text{ for } \frac{k(k+1)}{2} < n \le \frac{(k+1)(k+2)}{2} \end{cases}\] We already encountered that sequence here. We define \(g_n : [0,1] \to [0,1]\) by \[
g_n(x)=\begin{cases}
c(x) &\text{ for } n= 1\\
c \left(\frac{k+2}{2}(x-r_n) \right) &\text{ for } \frac{k(k+1)}{2} < n \le \frac{(k+1)(k+2)}{2} \end{cases}\] All the \(g_n\) functions are continuous because \(c\) is continuous and we have for \(n > 1\)\[
0 \le \int_0^1 g_n(x) \ dx \le \int_{\mathbb R} c \left(\frac{k+2}{2}(x-r_n) \right) \ dx = \frac{8}{\pi (k+2)}\] Hence \[
\lim\limits_{n \to \infty} \int_0^1 g_n(x) \ dx = 0\] We’ll be done if we prove that for all \(x \in [0,1]\), \((g_n(x))\) diverges. The detailed proof is left to the reader. The idea being for \(N \in \mathbb N\) to find \(N < \frac{k(k+1)}{2} < n_1 \le \frac{(k+1)(k+2)}{2}\) such that \(g_{n_1}(x)=0\) and \(N < \frac{k(k+1)}{2} < n_2 \le \frac{(k+1)(k+2)}{2}\) such that \(g_{n_2}(x) \ge \frac{\sqrt 2}{2}\).