Most of Cauchy existence theorems for a differential equation
x′=f(t,x) where t is a real variable and x a vector on a real vectorial space E are valid when E is of finite dimension or a Banach space. This is however not true for the Peano existence theorem.
Take for E the space of real sequences converging to 0 for the norm ‖x‖=supn|xn|. For all x∈E let’s note y=f(x) the sequence (yn) defined by:
yn=√|xn|+1n+1 As limn→+∞yn=0, y belongs to E. As the real function √|x| is uniformly continuous on R, the function y=f(x) is continuous on E.
However, we’ll prove that the differential equation
x′=f(x) has no solution in E with initial condition x(0)=0. If u was a solution, there would exist a sequence u(t)=[un(t)] of differentiable functions in a neighborhood of 0 satisfying:
u′n(t)=√|un(t)|+1n+1 and un(0)=0 for all n∈N.
By direct integration it can be proved that each equation (3) has a unique solution satisfying un(0)=0. For all n∈N, un is an odd function defined on all R and verifying for t≥0: un(t)≥t24. This last inequality, valid for all n∈N contradicts the ability for u to belongs to E. Therefore, the equation (2) has no solution.
This counterexample is from the French mathematician Jean Dieudonné.

Dear Jean-Pierre Merx,
I done my calculations again and now I see that I was mistaken. Sorry for the trouble and thanks for providing the proof, now the inequality seems clear to me.
Best regards,
Tadashi
Dear Jean-Pierre Merx, congratulations for the wonderful site!
I wish success for the site and I’m looking forward for more counterexamples
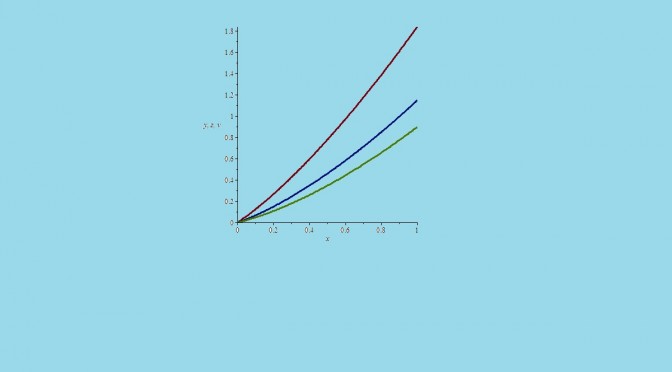
Between, I think there is a mistake in the last inequality of this counterexample, i.e. u_n(t) ≥ t ²/4 for t ≥ 0.
Consider for instance that n = 2. Then as the following plot suggests, there exists a q ≥ 0 such that the inequality is not valid for all t ≥ q.
Is there another way to prove that u doesn’t belongs to E?
Thanks a lot and best regards!
Dear Tadashi,
Thanks you very much for your encouragement! Indeed, I intend to publish more content. My target is at least a post per week, or more if I have more time.
In fact the inequality un(t)≥t24 can be proven either by direction integration or by considering the inequality u′n(t)>√un(t) valid for all t>0.
I have an issue to go to the link you provided. If you post it again, maybe I can have a look at it.
Thanks, Jean-Pierre.