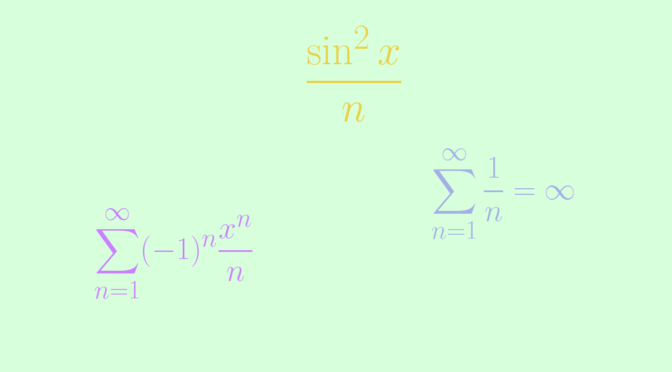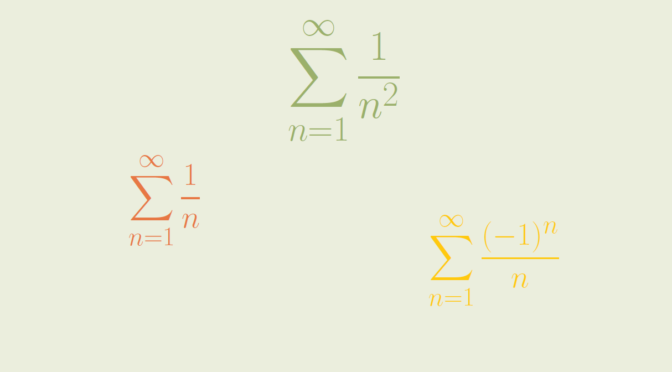Category Archives: Analysis

Analysis

A uniformly but not normally convergent function series

Leave a comment

Consider a functions series \(\displaystyle \sum f_n\) of functions defined on a set \(S\) to \(\mathbb R\) or \(\mathbb C\). It is known that if \(\displaystyle \sum f_n\) is normally convergent, then \(\displaystyle \sum f_n\) is uniformly convergent.

The converse is not true and we provide two counterexamples.

Consider first the sequence of functions \((g_n)\) defined on \(\mathbb R\) by:
\[g_n(x) = \begin{cases}
\frac{\sin^2 x}{n} & \text{for } x \in (n \pi, (n+1) \pi)\\
0 & \text{else}
\end{cases}\] The series \(\displaystyle \sum \Vert g_n \Vert_\infty\) diverges as for all \(n \in \mathbb N\), \(\Vert g_n \Vert_\infty = \frac{1}{n}\) and the harmonic series \(\sum \frac{1}{n}\) diverges. However the series \(\displaystyle \sum g_n\) converges uniformly as for \(x \in \mathbb R\) the sum \(\displaystyle \sum g_n(x)\) is having only one term and \[
\vert R_n(x) \vert = \left\vert \sum_{k=n+1}^\infty g_k(x) \right\vert \le \frac{1}{n+1}\]

For our second example, we consider the sequence of functions \((f_n)\) defined on \([0,1]\) by \(f_n(x) = (-1)^n \frac{x^n}{n}\). For \(x \in [0,1]\) \(\displaystyle \sum (-1)^n \frac{x^n}{n}\) is an alternating series whose absolute value of the terms converge to \(0\) monotonically. According to Leibniz test, \(\displaystyle \sum (-1)^n \frac{x^n}{n}\) is well defined and we can apply the classical inequality \[
\displaystyle \left\vert \sum_{k=1}^\infty (-1)^k \frac{x^k}{k} – \sum_{k=1}^m (-1)^k \frac{x^k}{k} \right\vert \le \frac{x^{m+1}}{m+1} \le \frac{1}{m+1}\] for \(m \ge 1\). Which proves that \(\displaystyle \sum (-1)^n \frac{x^n}{n}\) converges uniformly on \([0,1]\).

However the convergence is not normal as \(\sup\limits_{x \in [0,1]} \frac{x^n}{n} = \frac{1}{n}\).

Analysis

Root test

Leave a comment

The root test is a test for the convergence of a series \[
\sum_{n=1}^\infty a_n \] where each term is a real or complex number. The root test was developed first by Augustin-Louis Cauchy.

We denote \[l = \limsup\limits_{n \to \infty} \sqrt[n]{\vert a_n \vert}.\] \(l\) is a non-negative real number or is possibly equal to \(\infty\). The root test states that:

  • if \(l < 1\) then the series converges absolutely;
  • if \(l > 1\) then the series diverges.

The root test is inconclusive when \(l = 1\).

A case where \(l=1\) and the series diverges

The harmonic series \(\displaystyle \sum_{n=1}^\infty \frac{1}{n}\) is divergent. However \[\sqrt[n]{\frac{1}{n}} = \frac{1}{n^{\frac{1}{n}}}=e^{- \frac{1}{n} \ln n} \] and \(\limsup\limits_{n \to \infty} \sqrt[n]{\frac{1}{n}} = 1\) as \(\lim\limits_{n \to \infty} \frac{\ln n}{n} = 0\).

A case where \(l=1\) and the series converges

Consider the series \(\displaystyle \sum_{n=1}^\infty \frac{1}{n^2}\). We have \[\sqrt[n]{\frac{1}{n^2}} = \frac{1}{n^{\frac{2}{n}}}=e^{- \frac{2}{n} \ln n} \] Therefore \(\limsup\limits_{n \to \infty} \sqrt[n]{\frac{1}{n^2}} = 1\), while the series \(\displaystyle \sum_{n=1}^\infty \frac{1}{n^2}\) is convergent as we have seen in the ratio test article. Continue reading Root test →

Analysis

Ratio test

1 Comment

The ratio test is a test for the convergence of a series \[
\sum_{n=1}^\infty a_n \] where each term is a real or complex number and is nonzero when \(n\) is large. The test is sometimes known as d’Alembert’s ratio test.

Suppose that \[\lim\limits_{n \to \infty} \left\vert \frac{a_{n+1}}{a_n} \right\vert = l\] The ratio test states that:

  • if \(l < 1\) then the series converges absolutely;
  • if \(l > 1\) then the series diverges.

What if \(l = 1\)? One cannot conclude in that case.

Cases where \(l=1\) and the series diverges

Consider the harmonic series \(\displaystyle \sum_{n=1}^\infty \frac{1}{n}\). We have \(\lim\limits_{n \to \infty} \frac{n+1}{n} = 1\). It is well know that the harmonic series diverges. Recall that one proof uses the Cauchy’s convergence test based for \(k \ge 1\) on the inequalities: \[
\sum_{n=2^k+1}^{2^{k+1}} \frac{1}{n} \ge \sum_{n=2^k+1}^{2^{k+1}} \frac{1}{2^{k+1}} = \frac{2^{k+1}-2^k}{2^{k+1}} \ge \frac{1}{2}\]

An even simpler case is the series \(\displaystyle \sum_{n=1}^\infty 1\).

Cases where \(l=1\) and the series converges

We also have \(\lim\limits_{n \to \infty} \left\vert \frac{a_{n+1}}{a_n} \right\vert = 1\) for the infinite series \(\displaystyle \sum_{n=1}^\infty \frac{1}{n^2}\). The series is however convergent as for \(n \ge 1\) we have:\[
0 \le \frac{1}{(n+1)^2} \le \frac{1}{n(n+1)} = \frac{1}{n} – \frac{1}{n+1}\] and the series \(\displaystyle \sum_{n=1}^\infty \left(\frac{1}{n} – \frac{1}{n+1} \right)\) obviously converges.

Another example is the alternating series \(\displaystyle \sum_{n=1}^\infty \frac{(-1)^n}{n}\).

Analysis

A continuous function with divergent Fourier series

1 Comment

It is known that for a piecewise continuously differentiable function \(f\), the Fourier series of \(f\) converges at all \(x \in \mathbb R\) to \(\frac{f(x^-)+f(x^+)}{2}\).

We describe Fejér example of a continuous function with divergent Fourier series. Fejér example is the even, \((2 \pi)\)-periodic function \(f\) defined on \([0,\pi]\) by: \[
f(x) = \sum_{p=1}^\infty \frac{1}{p^2} \sin \left[ (2^{p^3} + 1) \frac{x}{2} \right]\]
According to Weierstrass M-test, \(f\) is continuous. We denote \(f\) Fourier series by \[
\frac{1}{2} a_0 + (a_1 \cos x + b_1 \sin x) + \dots + (a_n \cos nx + b_n \sin nx) + \dots.\]

As \(f\) is even, the \(b_n\) are all vanishing. If we denote for all \(m \in \mathbb N\):\[
\lambda_{n,m}=\int_0^{\pi} \sin \left[ (2m + 1) \frac{t}{2} \right] \cos nt \ dt \text{ and } \sigma_{n,m} = \sum_{k=0}^n \lambda_{k,m},\]
we have:\[
\begin{aligned}
a_n &=\frac{1}{\pi} \int_{-\pi}^{\pi} f(t) \cos nt \ dt= \frac{2}{\pi} \int_0^{\pi} f(t) \cos nt \ dt\\
&= \frac{2}{\pi} \int_0^{\pi} \left(\sum_{p=1}^\infty \frac{1}{p^2} \sin \left[ (2^{p^3} + 1) \frac{x}{2} \right]\right) \cos nt \ dt\\
&=\frac{2}{\pi} \sum_{p=1}^\infty \frac{1}{p^2} \int_0^{\pi} \sin \left[ (2^{p^3} + 1) \frac{x}{2} \right] \cos nt \ dt\\
&=\frac{2}{\pi} \sum_{p=1}^\infty \frac{1}{p^2} \lambda_{n,2^{p^3-1}}
\end{aligned}\] One can switch the \(\int\) and \(\sum\) signs as the series is normally convergent.

We now introduce for all \(n \in \mathbb N\):\[
S_n = \frac{\pi}{2} \sum_{k=0}^n a_k = \sum_{p=1}^\infty \sum_{k=0}^n \frac{1}{p^2} \lambda_{k,2^{p^3-1}}
=\sum_{p=1}^\infty \frac{1}{p^2} \sigma_{n,2^{p^3-1}}\]

We will prove below that for all \(n,m \in \mathbb N\) we have \(\sigma_{m,m} \ge \frac{1}{2} \ln m\) and \(\sigma_{n,m} \ge 0\). Assuming those inequalities for now, we get:\[
S_{2^{p^3-1}} \ge \frac{1}{p^2} \sigma_{2^{p^3-1},2^{p^3-1}} \ge \frac{1}{2p^2} \ln(2^{p^3-1}) = \frac{p^3-1}{2p^2} \ln 2\]
As the right hand side diverges to \(\infty\), we can conclude that \((S_n)\) diverges and consequently that the Fourier series of \(f\) diverges at \(0\). Continue reading A continuous function with divergent Fourier series →

Analysis

Radius of convergence of power series

Leave a comment

We look here at the radius of convergence of the sum and product of power series.

Let’s recall that for a power series \(\displaystyle \sum_{n=0}^\infty a_n x^n\) where \(0\) is not the only convergence point, the radius of convergence is the unique real \(0 < R \le \infty\) such that the series converges whenever \(\vert x \vert < R\) and diverges whenever \(\vert x \vert > R\).

Given two power series with radii of convergence \(R_1\) and \(R_2\), i.e.
\begin{align*}
\displaystyle f_1(x) = \sum_{n=0}^\infty a_n x^n, \ \vert x \vert < R_1 \\ \displaystyle f_2(x) = \sum_{n=0}^\infty b_n x^n, \ \vert x \vert < R_2 \end{align*} The sum of the power series \begin{align*} \displaystyle f_1(x) + f_2(x) &= \sum_{n=0}^\infty a_n x^n + \sum_{n=0}^\infty b_n x^n \\ &=\sum_{n=0}^\infty (a_n + b_n) x^n \end{align*} and its Cauchy product:
\begin{align*}
\displaystyle f_1(x) \cdot f_2(x) &= \left(\sum_{n=0}^\infty a_n x^n\right) \cdot \left(\sum_{n=0}^\infty b_n x^n \right) \\
&=\sum_{n=0}^\infty \left( \sum_{l=0}^n a_l b_{n-l}\right) x^n
\end{align*}
both have radii of convergence greater than or equal to \(\min \{R_1,R_2\}\).

The radii can indeed be greater than \(\min \{R_1,R_2\}\). Let’s give examples.
Continue reading Radius of convergence of power series →

Analysis

Counterexample around Morera’s theorem

Leave a comment

Let’s recall Morera’s theorem.

Morera’s theorem Suppose that \(f\) is a continuous complex-valued function in a connected open set \(\Omega \subset \mathbb C\) such that
\[\int_{\partial \Delta} f(z) \ dz = 0\] for every closed triangle \(\Delta \subset \Omega \setminus \{p\}\) where \(p \in \Omega\). Then \(f\) is holomorphic in \(\Omega\).

Does the conclusion of Morera’s theorem still hold if \(f\) is supposed to be continuous only in \(\Omega \setminus \{p\}\)? The answer is negative and we provide a counterexample.

Let \(\Omega\) be the entire complex plane, \(f\) defined as follows
\[f(z)=\begin{cases}
\frac{1}{z^2} & \text{if } z \neq 0\\
0 & \text{otherwise}
\end{cases}\] and \(p\) the origin.

For \(a,b \in \Omega \setminus \{0\}\) we have
\[\begin{aligned}
\int_{[a,b]} f(z) \ dz &= \int_{[a,b]} \frac{dz}{z^2}\\
&= \int_0^1 \frac{b-a}{[a+t(b-a)]^2} \ dt\\
&=\left[ -\frac{1}{a+t(b-a)} \right]_0^1 = \frac{1}{a} – \frac{1}{b}
\end{aligned}\]

Hence for a triangle \(\Delta\) with vertices at \(a,b ,c \in \Omega \setminus \{0\}\):
\[\int_{\partial \Delta} f(z) \ dz = \left( \frac{1}{a} – \frac{1}{b} \right) + \left( \frac{1}{b} – \frac{1}{c} \right) + \left( \frac{1}{c} – \frac{1}{a} \right)=0\]

However, \(f\) is not holomorphic in \(\Omega\) as it is even not continuous at \(0\).

Analysis

Continuity versus uniform continuity

Leave a comment

We consider real-valued functions.

A real-valued function \(f : I \to \mathbb R\) (where \(I \subseteq\) is an interval) is continuous at \(x_0 \in I\) when: \[(\forall \epsilon > 0) (\exists \delta > 0)(\forall x \in I)(\vert x- x_0 \vert \le \delta \Rightarrow \vert f(x)- f(x_0) \vert \le \epsilon).\] When \(f\) is continuous at all \(x \in I\), we say that \(f\) is continuous on \(I\).

\(f : I \to \mathbb R\) is said to be uniform continuity on \(I\) if \[(\forall \epsilon > 0) (\exists \delta > 0)(\forall x,y \in I)(\vert x- y \vert \le \delta \Rightarrow \vert f(x)- f(y) \vert \le \epsilon).\]

Obviously, a function which is uniform continuous on \(I\) is continuous on \(I\). Is the converse true? The answer is negative.

An (unbounded) continuous function which is not uniform continuous

The map \[
\begin{array}{l|rcl}
f : & \mathbb R & \longrightarrow & \mathbb R \\
& x & \longmapsto & x^2 \end{array}\] is continuous. Let’s prove that it is not uniform continuous. For \(0 < x < y\) we have \[\vert f(x)-f(y) \vert = y^2-x^2 = (y-x)(y+x) \ge 2x (y-x)\] Hence for \(y-x= \delta >0\) and \(x = \frac{1}{\delta}\) we get
\[\vert f(x) -f(y) \vert \ge 2x (y-x) =2 > 1\] which means that the definition of uniform continuity is not fulfilled for \(\epsilon = 1\).

For this example, the function is unbounded as \(\lim\limits_{x \to \infty} x^2 = \infty\). Continue reading Continuity versus uniform continuity →

Analysis

Continuity under the integral sign

Leave a comment

We consider here a measure space \((\Omega, \mathcal A, \mu)\) and \(T \subset \mathbb R\) a topological subspace. For a map \(f : T \times \Omega \to \mathbb R\) such that for all \(t \in T\) the map \[
\begin{array}{l|rcl}
f(t, \cdot) : & \Omega & \longrightarrow & \mathbb R \\
& \omega & \longmapsto & f(t,\omega) \end{array}
\] is integrable, one can define the function \[
\begin{array}{l|rcl}
F : & T & \longrightarrow & \mathbb R \\
& t & \longmapsto & \int_\Omega f(t,\omega) \ d\mu(\omega) \end{array}
\]

Following theorem is well known (and can be proven using dominated convergence theorem):

THEOREM for an adherent point \(x \in T\), if

  • \(\forall \omega \in \Omega \lim\limits_{t \to x} f(t,\omega) = \varphi(\omega)\)
  • There exists a map \(g : \Omega \to \mathbb R\) such that \(\forall t \in T, \, \forall \omega \in \Omega, \ \vert f(t,\omega) \vert \le g(\omega)\)

then \(\varphi\) is integrable and \[
\lim\limits_{t \to x} F(t) = \int_\Omega \varphi(\omega) \ d\mu(\omega)\]
In other words, one can switch \(\lim\) and \(\int\) signs.

We provide here a counterexample showing that the conclusion of the theorem might not hold if \(f\) is not bounded by a function \(g\) as supposed in the premises of the theorem. Continue reading Continuity under the integral sign →

Analysis

A trigonometric series that is not a Fourier series (Lebesgue-integration)

Leave a comment

We already provided here an example of a trigonometric series that is not the Fourier series of a Riemann-integrable function (namely the function \(\displaystyle x \mapsto \sum_{n=1}^\infty \frac{\sin nx}{\sqrt n}\)).

Applying an Abel-transformation (like mentioned in the link above), one can see that the function \[f(x)=\sum_{n=2}^\infty \frac{\sin nx}{\ln n}\] is everywhere convergent. We now prove that \(f\) cannot be the Fourier series of a Lebesgue-integrable function. The proof is based on the fact that for a \(2 \pi\)-periodic function \(g\), Lebesgue-integrable on \([0,2 \pi]\), the sum \[\sum_{n=1}^\infty \frac{c_n-c_{-n}}{n}\] is convergent where \((c_n)_{n \in \mathbb Z}\) are the complex Fourier coefficients of \(g\): \[c_n = \frac{1}{2 \pi} \int_0^{2 \pi} g(t)e^{-ikt} \ dt.\] As the series \(\displaystyle \sum_{n=2}^\infty \frac{1}{n \ln n}\) is divergent, we will be able to conclude that the sequence defined by \[\gamma_0=\gamma_1=\gamma_{-1} = 0, \, \gamma_n=- \gamma_{-n} = \frac{1}{\ln n} \ (n \ge 2)\] cannot be the Fourier coefficients of a Lebesgue-integrable function, hence that \(f\) is not the Fourier series of any Lebesgue-integrable function. Continue reading A trigonometric series that is not a Fourier series (Lebesgue-integration) →

Analysis

Playing with liminf and limsup

Leave a comment

Let’s consider real sequences \((a_n)_{n \in \mathbb N}\) and \((b_n)_{n \in \mathbb N}\). We look at inequalities involving limit superior and limit inferior of those sequences. Following inequalities hold:
\[\begin{aligned}
& \liminf a_n + \liminf b_n \le \liminf (a_n+b_n)\\
& \liminf (a_n+b_n) \le \liminf a_n + \limsup b_n\\
& \liminf a_n + \limsup b_n \le \limsup (a_n+b_n)\\
& \limsup (a_n+b_n) \le \limsup a_n + \limsup b_n
\end{aligned}\] Let’s prove for example the first inequality, reminding first that \[
\liminf\limits_{n \to \infty} a_n = \lim\limits_{n \to \infty} \left(\inf\limits_{m \ge n} a_m \right).\] For \(n \in \mathbb N\), we have for all \(m \ge n\) \[\inf\limits_{k \ge n} a_k + \inf\limits_{k \ge n} b_k \le a_m + b_m\] hence \[\inf\limits_{k \ge n} a_k + \inf\limits_{k \ge n} b_k \le \inf\limits_{k \ge n} \left(a_k+b_k \right)\] As the sequences \((\inf\limits_{k \ge n} a_k)_{n \in \mathbb N}\) and \((\inf\limits_{k \ge n} b_k)_{n \in \mathbb N}\) are non-increasing we get for all \(n \in \mathbb N\), \[\liminf a_n + \liminf b_n \le \inf\limits_{m \ge n} \left(a_m+b_m \right)\] which leads finally to the desired inequality \[\liminf a_n + \liminf b_n \le \liminf (a_n+b_n).\] Continue reading Playing with liminf and limsup →