In that article, I described some properties of Thomae’s function\(f\). Namely:

• The function is discontinuous on \(\mathbb{Q}\).
• Continuous on \(\mathbb{R} \setminus \mathbb{Q}\).
• Its right-sided and left-sided limits vanish at all points.

Let’s modify \(f\) to get function \(g\) defined as follow:
\[g:
\left|\begin{array}{lrl}
\mathbb{R} & \longrightarrow & \mathbb{R} \\
x & \longmapsto & 0 \text{ if } x \in \mathbb{R} \setminus \mathbb{Q} \\
\frac{p}{q} & \longmapsto & q \text{ if } \frac{p}{q} \text{ in lowest terms and } q > 0
\end{array}\right.\] \(f\) and \(g\) both vanish on the set of irrational numbers, while on the set of rational numbers, \(g\) is equal to the reciprocal of \(f\). We now consider an open subset \(O \subset \mathbb{R}\) and \(x \in O\). As \(f\) right-sided and left-sided limits vanish at all points, we have \(\lim\limits_{n \to +\infty} f(x_n) = 0\) for all sequence \((x_n)\) of rational numbers converging to \(x\) (and such a sequence exists as the rational numbers are everywhere dense in the reals). Hence \(\lim\limits_{n \to +\infty} g(x_n) = + \infty\) as \(f\) is positive.

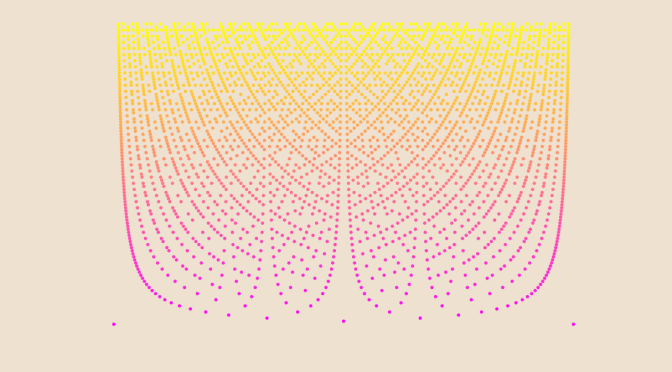
We can conclude that \(g\) is nowhere locally bounded. The picture of the article is a plot of function \(g\) on the rational numbers \(r = \frac{p}{q}\) in lowest terms for \(0 < r < 1\) and \(q \le 50\).