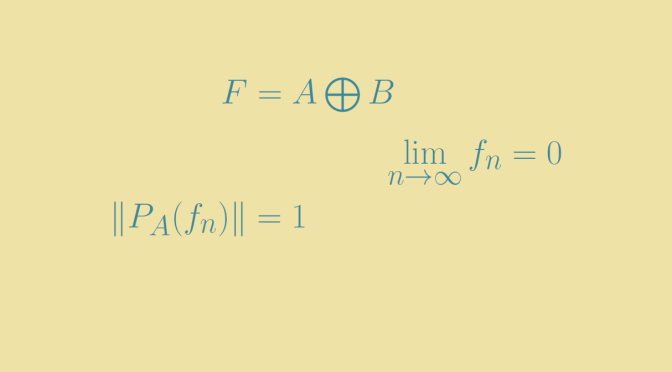We give here an example of a two complemented subspaces $A$ and $B$ that are not topologically complemented.

For this, we consider a vector space of infinite dimension equipped with an inner product. We also suppose that $E$ is separable. Hence, $E$ has an orthonormal basis $(e_n)_{n \in \mathbb N}$.

Let $a_n=e_{2n}$ and $b_n=e_{2n}+\frac{1}{2n+1} e_{2n+1}$. We denote $A$ and $B$ the closures of the linear subspaces generated by the vectors $(a_n)$ and $(b_n)$ respectively. We consider $F=A+B$ and prove that $A$ and $B$ are complemented subspaces in $F$, but not topologically complemented.

$A$ and $B$ are complemented subspaces in $F$

Let $\langle \cdot , \cdot \rangle$ be the inner product. For $i \neq j$ integers we have $\langle a_i, a_j \rangle =0$ and $\langle b_i, b_j \rangle =0$ as $(e_n)_{n \in \mathbb N}$ is an orthonormal basis. Therefore all $x \in A$ can be written $\displaystyle \sum_{n=0}^{+\infty} \langle x , e_{2n} \rangle e_{2n}$ and all $x \in B$ can be written $\displaystyle \sum_{n=0}^{+\infty} \langle x , b_n \rangle \frac{b_n}{\Vert b_n \Vert^2}$.

If $x \in A \cap B$, we have for all $n \in \mathbb N$ $\langle x, e_{2n+1} \rangle =0$ as $x \in A$. Hence $$x=\sum_{n=0}^{+\infty} \langle x , e_{2n} \rangle \frac{b_n}{\Vert b_n \Vert^2}$$ Making the inner product of $x$ with $e_{2n+1}$ we get $$\langle x, e_{2n+1} \rangle = \langle x , e_{2n} \rangle \frac{\Vert e_{2n+1} \Vert^2}{(2n+1)\Vert b_n \Vert^2} = 0$$ Consequently, we have $\langle x, e_{2n} \rangle$ for all $n \in \mathbb N$ and finally $x = 0$ which proves that $A$ and $B$ are complemented subspaces in $F$.

$A$ and $B$ are not topologically complemented

Let $(x_n)$ be the sequence $x_n = b_n-a_n$. We have $x_n=\frac{e_{2n+1}}{n+1}$ and $\lim\limits_{n \to \infty} x_n = 0$. If $A$ and $B$ were topologically complemented, the projection of $F$ on the subspace $A$ would be continuous. But the projection of $x_n$ is $a_n$ as $A$ and $B$ are complemented subspaces. However $\Vert a_n \Vert = 1$ for all $n \in \mathbb N$ as $(e_n)$ is an orthonormal basis. A contradiction proving that $A$ and $B$ are not topologically complemented.