We consider here sequences of real functions defined on a closed interval. Following theorem is the main one regarding the differentiation of the limit.
Theorem: Suppose \((f_n)\) is a sequence of functions, differentiable on \([a,b]\) and such that \((f_n(x_0))\) converges for some point \(x_0 \in [a,b]\). If \((f_n^\prime)\) converges uniformly on \([a,b]\), then \((f_n)\) converges uniformly on \([a,b]\) to a function \(f\) and for all \(x \in [a,b]\) \[f^\prime(x)=\lim\limits_{n \to \infty} f_n^\prime(x)\] What happens if we drop some hypothesis of the theorem?
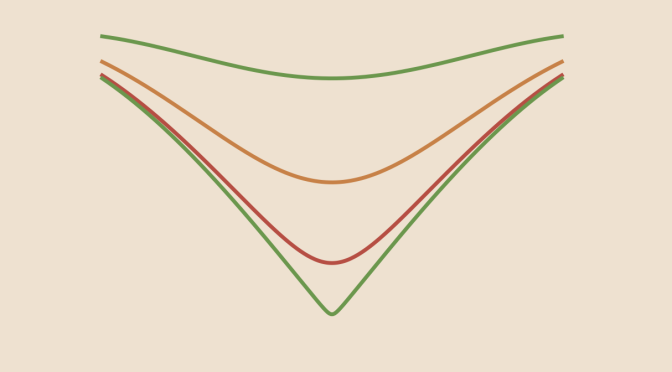
If \((f_n)\) converges uniformly to \(f\), and the functions \(f_n\) are differentiable, does this imply that \(f\) is also differentiable?
The answer is no. An example is the sequence of functions
\[\begin{array}{l|rcl}
f_n : & [-1,1] & \longrightarrow & \mathbb{R} \\
& x & \longmapsto & \sqrt{\frac{1}{n^2}+x^2} \end{array}\] These functions are differentiable according to the chain rule as \(g_n(x): x \longmapsto \frac{1}{n^2}+x^2\) is strictly positive on \([-1,1]\). We also have \[x^2 \le f_n^2(x) = \frac{1}{n^2}+x^2 \le (\vert x \vert +\frac{1}{n})^2\] hence
\[\vert x \vert \le f_n(x) \le \vert x \vert +\frac{1}{n}\] as \(f_n\) is positive. So by the squeeze test, \((f_n)\) converges uniformly to the absolute value function \(f(x) = \vert x \vert\). But this function is not differentiable at \(0\). Thus, the uniform limit of differentiable functions need not be differentiable.
If \((f_n)\) converges uniformly to \(f\), and the functions \(f_n\) are differentiable, does \((f_n^\prime)\) also converges?
The answer is again negative as we can see considering the functions \[\begin{array}{l|rcl}
f_n : & [0,2 \pi] & \longrightarrow & \mathbb{R} \\
& x & \longmapsto & \frac{\sin nx}{\sqrt{n}} \end{array}\] As \(\vert \sin y \vert \le 1\) for \(y \in \mathbb{R}\) we get for \(x \in [0,2 \pi]\)
\[0 \le \vert f_n(x) \vert \le \frac{1}{\sqrt{n}}\] Hence \((f_n)\) converges uniformly to the always vanishing function \(f(x)=0\). On the other hand, \(f_n^\prime(x)=\sqrt{n} \cos nx\). And so in particular \(f_n^\prime(0)=\sqrt{n}\) does not converge. Consequently, \((f_n^\prime)\) does not converge pointwise (nor uniformly).
And if \((f_n^\prime)\) converges, is the limit \(f^\prime\)?
Again not. Consider \[\begin{array}{l|rcl}
f_n : & [-1,1] & \longrightarrow & \mathbb{R} \\
& x & \longmapsto & \frac{x}{1+n^2 x^2} \end{array}\] On one hand, we have for all \(x \in [-1,1]\), \(\vert f_n(x) \vert \le \vert x \vert \) as \(1+n^2 x^2 > 1\). Hence \(\vert f_n(x) \vert \le \frac{1}{n}\) for \(\vert x \vert \le \frac{1}{n}\).
On the other hand for \(\vert x \vert \ge \frac{1}{n}\), \(n^2 \vert x \vert \ge n\) therefore
\[\vert f_n(x) \vert \le \frac{1}{n^2 \vert x \vert} \le \frac{1}{n}\] Finally for all \(x \in [-1,1]\) \[\vert f_n(x) \vert \le \frac{1}{n}\] and \((f_n)\) converges uniformly to the function \(f(x)=0\) using the squeeze test.
\(f_n\) is differentiable with
\[f_n^\prime(x) = \frac{1-n x^2}{(1+ n x^2)^2}\] For all \(n \ge 1\), \(f_n^\prime(0) = 1\) and for \(x \neq 0\)
\[\vert f_n^\prime(x) \vert \le \frac{1+n x^2}{(1+ n x^2)^2} = \frac{1}{1+ n x^2} \underset{n \to \infty}{\to} 0\] Conclusion: \((f_n)\) is a sequence of differentiable functions that converges uniformly to the zero function whose sequence of derivatives converges to a function different that the derivative of the limit.