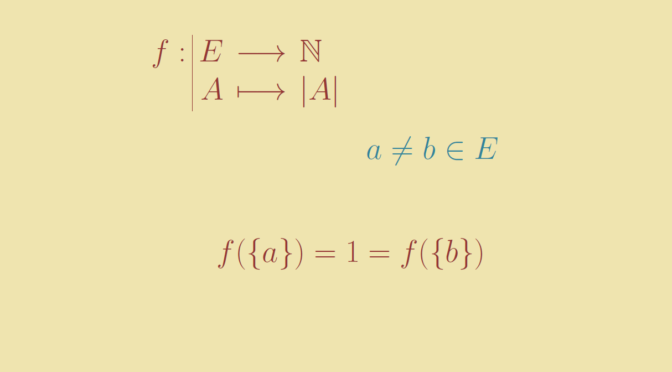Consider two partially ordered sets $(E,\le)$ and $(F,\le)$ and a strictly increasing map $f : E \to F$. If the order $(E,\le)$ is total, then $f$ is one-to-one. Indeed for distinct elements $x,y \in E$, we have either $x < y$ or $y < x$ and consequently $f(x) < f(y)$ or $f(y) < f(x)$. Therefore $f(x)$ and $f(y)$ are different. This is not true anymore for a partial order $(E,\le)$. We give a counterexample.

Consider a finite set $E$ having at least two elements and partially ordered by the inclusion. Let $f$ be the map defined on the powerset $\wp(E)$ that maps $A \subseteq E$ to its cardinal $\vert A \vert$. $f$ is obviously strictly increasing. However $f$ is not one-to-one as for distincts elements $a,b \in E$ we have $$f(\{a\}) = 1 = f(\{b\})$$